ELECTROSTATICS ASSIGNMENT AND SOLUTIONS

Problem 1:

What is the net force and its direction that the charges at the vertices A and C of the right triangle ABC exert on the charge in vertex B?

Solution to Problem 1:

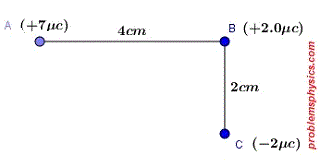
Let F_AB be the force of repulsion exerted by the charge at A on the charge at B and F_CB be the force exerted by the charge at point C on the charge at point B. The diagram below shows the direction of these two forces. We first use Coulomb’s law (F = k q1 q2 / r²) to find the magnitude of these two forces

|F_AB| = k (7 × 10^-6)((2× 10^-6) / (4 × 10^-2)² = 14 × 10^-12 k / (16 × 10^-4) = 0.875 k × 10^-8

|F_CB| = k (2× 10^-6)(2× 10^-6) / (2 × 10^-2)² = k × 10^-8

We now use Pythagora’s theorem to find the magnitude of the resultant force F = F_AB + F_CB (a vector sum)

|F| = √(|F_AB|² + |F_CB|²) = k × 10^-8 √( 0.875 ² + 1² ) = 9.00 × 10⁹ × 10^-8 √( 0.875 ² + 1² ) = 1.20 × 10² N

θ = arctan(|F_CB|/ |F_AB| ) = arctan( k × 10^-8 / 0.875 k × 10^-8) = 48.8°

---

Problem 2:

A positive charge q exerts a force of magnitude – 0.20 N on another charge – 2q. Find the magnitude of each charge if the distance separating them is equal to 50 cm.

Solution to Problem 2:

The force that q exert on 2q is given by Coulomb’s law:

F = k (q) ( – 2q) / r² , r = 0.5 m , F = – 0.20 N ,

– 0.2 = – 2 q² k / 0.5²

q² = 0.2 × 0.5² / (2 k)

q = √ [ (0.2 × 0.5² / (2 × 9 × 10⁹) ] = 1.66 × 10^-6 C

q = 1.66 × 10^-6 C , -2 q = -3.23 × 10^-6 C

---

Problem 3:

Two identical objects, separated by a distance d, with charges equal in magnitude but of opposite signs exert a force of attraction of – 2.5 N on each other. What force do these objects exert on each other if the distance between them becomes 2d?

Solution to Problem 3:

Let the two charges be q and -q. The magnitude of the force that q and -q, separated by a distance d, exert on each other is given by Coulomb’s law:

F = k (q) (- q) / d² = – k q² / d² = – 2.5 N

The magnitude of the force F₂ that q and -q, separated by a distance 2 d, exert on each other is given by Coulomb’s law:

F₂ = k (q) (- q) / (2 d)² = – k q² / 4 d² = F / 4 = – 2.5 / 4 = – 0.625 N

---

Problem 4:

A charge of q = – 4.0 × 10^-6 is placed in an electric field and experiences a force of 5.5 N [E]

a) What is the magnitude and direction of the electric field at the point where charge q is located?

b) If charge q is removed, what is the magnitude and direction of the force exerted on a charge of – 2q at the same location as charge q?

Solution to Problem 4:

a) The force on a charge q due to an electric field E is given by

F = q E

The magnitude of E is given by

| E | = | F | / | q | = 5.5 / (4.0 × 10^-6) = 1.375 × 10⁶ N / C

Since charge q is negative F and E have opposite direction. E is 1.375 × 10⁶ N / C [W]

b) The force on a charge -2q due to an electric field E is given by

F₂ = -2 q E = -2(q E) = -2(5.5[E]) = -11 [E] or 11 N [W]

---

Problem 5:

Three charges are located at the vertices of a right isosceles triangle as shown below. What is the magnitude and direction of the resultant electric field at the midpoint M of AC?

Solution to Problem 5:

The magnitude of an electric field due to a charge q is given by.

E = k q / r²

and it is directed away from charge q if q is positive and towards charge q if q is negative. Hence the diagram below showing the direction the fields due to all the three charges. The total field field E is the vector sum of all three fields: E_AM, E_CM and E_BM

E = E_AM + E_CM + E_BM (vector sum)

Because of the symmetry at point M, E_AM and E_CM are equal in magnitudes and opposite directions. Hence

E_AM + E_CM = 0 (vector addition)

Hence

E = E_BM

The magnitude of E_BM is given by

E_BM = k (2 × 10^-6) / BM²

Note that

∠MBA = ∠MAB = 45° and MB = MA (lengths)

hence

MB = MA

Pythagora’s theorem

5² = MB² + MA²

MB = 5/√2

MB² = 25/2 = 12.5 cm² = 12.5×10^-4 m²

The magnitude of the field E_BM at point M due to the charge at B is given by

E_BM = k q / MB² = k (2 × 10^-6) / (12.5 × 10^-4) = 9.00 × 10⁹ × 2 × 10^-6 / (12.5 × 10^-4) = 1.44 × 10⁷ N/C

The magnitude of the total field E at M is equal to E_BM. Hence

E = E_BM = 1.44 × 10⁷ N/C

---

Problem 6:

What distance must separate two charges of + 5.6×10^-4C and -6.3×10^-4 C in order to have an electric potential energy with a magnitude of 5.0 J in the system of the two charges?

Solution to Problem 6:

The magnitude of the electric potiential energy E_p of a system of two charges q₁ and q₂ separated by a distance r is given by

E_p = k | q₁ | | q₂ | / r

Solve for r.

r = k q₁ q₂ / E_p = 9.00×10⁹×5.6×10^-4×6.3×10^-4 / 5.0 = 6.35×10² m

---

Problem 7:

The distance between two charges q₁ = + 2 μC and q₂ = + 6 μC is 15.0 cm. Calculate the distance from charge q₁ to the points on the line segment joining the two charges where the electric field is zero.

Solution to Problem 7:

At a distance x from q1 the total electric filed is the vector sum of the electric E₁ from due to q₁ and directed to the right and the electric field E₂ due to q₂ and directed to the left. The vector sum is equal to zero if the magnitudes of the the two fields E₁ and E₂ are equal since they have opposite direction.

The magnitudes of the the two fields E₁ and E₂ are given by

E₁ = k q1 / x²

E₂ = k q2 / (15 – x)²

E₁ = E₂ gives the equation

k q1 / x² = k q2 / (15 – x)²

Cross multiply and simplify to obtain

q1(15 – x)² = q2 x²

Which may be written as

(15 – x)² / x² = q2 / q1 = 3

We now to solve for x the equation

(15 – x) / x = ~+mn~ √3

The above equation gives two solutions but only one is positive and is equal to

x = 15 / (1 + √3) ≈ 5.50 cm

---

Problem 8:

The distance AB between charges Q1 and Q2 shown below is 5.0 m. How much work must be done to move charge Q2 to a new location at point C so that the distance BC = 2.5 m?

Solution to Problem 8:

If W is the work to be done to move Q2 from a position where its potential energy is E_p1 and kinetic energy 0 (from rest) to another position where its potential energy is E_p2 and kinetic energy 0 (to rest), then by the conservation of energy, we have.

E_p1 + W = E_p2

which gives

W = E_p2 – E_p1

E_p1 = k Q1 Q2 / AB , with AB = 5 m

E_p2 = k Q1 Q2 / AC , with AC = 7.5 m

W = k Q1 Q2 (1/AB – 1/AC) = 9.00×10⁹×5×10^-6×-3×10^-6 (1/7.5 – 1/5) = 9×10^-3J

---

Problem 9:

Two parallel plates separated by distance of 1 cm have a potential difference of 20 V between them. The plates are held in a horizontal position with the negative plate above the positive plate. An electron is released from rest in the upper plate.

a) What is the acceleration of the electron?

b)How long it takes to reach the lower plate?

c)What is the kinetic energy of the electron when it hits the lower plate?

Note: charge of electron q = -1.6×10^-19C , mass of electron m = 9.11×10^-31Kg

---

Solution to Problem 8:

a) Electric force F exerted on the electron is given by:

F = q E

with the electric field E between the plates given by:

E = ΔV / d = 20 v / 1 cm = 2000 v/m or N/C

F = -1.6×10^-19C×2000 N/C = -3.2×10^-16N

Note: the weight of the electron is given by: m g = 9.11×10^-31×9.8 = 8.93×10^-30N

which is much smaller that the electric force acting on the electron and will therefore be neglected.

The acceleration due to the electric force is:

a = F / m = -3.2×10^-16/9.11×10^-31 = – 3.51×10¹⁴ m/s²

b) Let v_f and v_i be the final (at lower plate) and initial (from rest upper plate ) velocities of the electron. Hence using the formula

v_f² = v_i² + 2 a h , h is the distance between the plates.

v_i = 0 (from rest)

v_f² = 2 a h = 2(- 3.51×10¹⁴ m/s²)(-1×10^-2m) (y axis upward)

v_f = 2.64×10⁶ m/s

With uniform acceleration a and initial velocity equal to zero, we have the velocity as a function of time given by

v = a t

If T is the time it takes the electron to move from the upper to the lower plates, then

v_f = a T

which gives

T = v_f / a = 2.64×10⁶ m/s / 3.51×10¹⁴ m/s² = 7.52×10^-9s

c) The kinetic energy E_k of the electron at the lower plate is given by

E_k = (1/2) m v_f² = 0.5×9.11×10^-31(2.64×10⁶)² = 3.17×10^-18J

---

Problem 10:

Two electrons are held 3μm apart. When released from rest, what is the velocity of each electron when they are 8μm apart?

Solution to Problem 10:

Let E_p1 be the potential electric energy at rest (distance r = 3μm) and E_p2 be the potential electric energy when they are 5μm apart and moving. The total (potential and kinetic energies) at each position are given by

E_t1 = E_p1 + (1/2) m (0)² = E_p1

E_t2 = E_p2 + (1/2) m v² + (1/2) m v² = E_p2 + m v²

Formula for electric potential energy due to charges q1 and q2 distant by r is:

E_p = k q1 q2 /r

No external energy is used and no energy is lost, therefore there is conservation of energy such that potential energy is converted into kinetic energy.

E_p1 = E_p2 + m v² , v is the velocity when 8μm apart.

charge of electron = – e = -1.60×10^-19C , mass of electrom m = 9.109×10^-31Kg

m v² = E_p1 – E_p2 = k×e×e / (3×10^-6) – k×e×e / (5×10^-6) = 9×10⁹(1.6×10^-19)² [ 1 / (3×10^-6) – 1 / (8×10^-6) ]

v ≈ 3.48×10⁴ m/s